Tolaso J Kos wrote:Use complex analysis methods to prove that: $$\int_{0}^{\infty}\frac{1-\cos x}{x^2}\,dx=\frac{\pi}{2}$$
Integrating by parts once we have that our integral equals to $\displaystyle \int_0^{\infty} \frac{\sin x}{x} \, {\rm d}x$. Due to the forum the exercise is I assume that we have to calculate the integral using complex analysis. For this purpose consider the function $\displaystyle f(z)=\frac{e^{iz}}{z}$ and integrate it on the counterclockwise contour presented below:
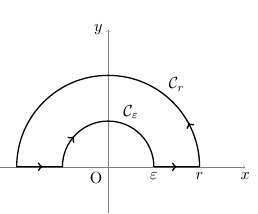
- contour.jpg (6.96 KiB) Viewed 3866 times
[/centre]
Now since no pole of $f$ is enclosed by the contour we have that $\displaystyle \ointctrclockwise f(z)\, {\rm d}z =0$. Splitting the contour apart to the components it is consisted of we have:
$$\oint_{\gamma}f(z)\, {\rm d}z = \int_{-R}^{\epsilon}f(x)\, {\rm d}x +
\oint_{\mathcal{C}_\epsilon}f(z)\, {\rm d}z + \int_{\epsilon}^{R}f(x)\, {\rm
d}x + \oint_{\mathcal{C}_R} f(z)\, {\rm d}z$$
The contribution of the small circle is actually $-i \pi$ , since:
$$\lim_{\epsilon \to 0} \oint_{\mathcal{C}_{\epsilon}}^{} \! \frac{e^{iz}}{z}
\, {\rm d}z =-i\lim_{\epsilon \to 0}\int_{-\pi}^0 \! e^{i\epsilon
\cos(t)+\epsilon \sin(t)} \, {\rm d}t =-i\int_{-\pi}^0 \! 1 \, {\rm
d}t=- i \pi$$
while the contribution of the large circle is $0$, because of:
$$\lim_{R\rightarrow +\infty}\left | \oint_{\mathcal{C}_R}\frac{e^{iz}}{z}\,
{\rm d}z \right | \leq \lim_{R\rightarrow
+\infty}\int_{0}^{\pi}\frac{{\rm d}t}{e^{R\sin t}}= \int_{0}^{\pi}0\,
{\rm d}t =0$$
Thus:
$$\int_{-\infty}^{0}\frac{e^{iz}}{z}\, {\rm d}z +
\int_{0}^{\infty}\frac{e^{iz}}{z}\, {\rm d}z= i \pi \Leftrightarrow
\int_{-\infty}^{\infty}\frac{e^{iz}}{z}\, {\rm d}z = i \pi$$
Taking the imaginary part and dividing by $\frac{1}{2}$ yields the result.